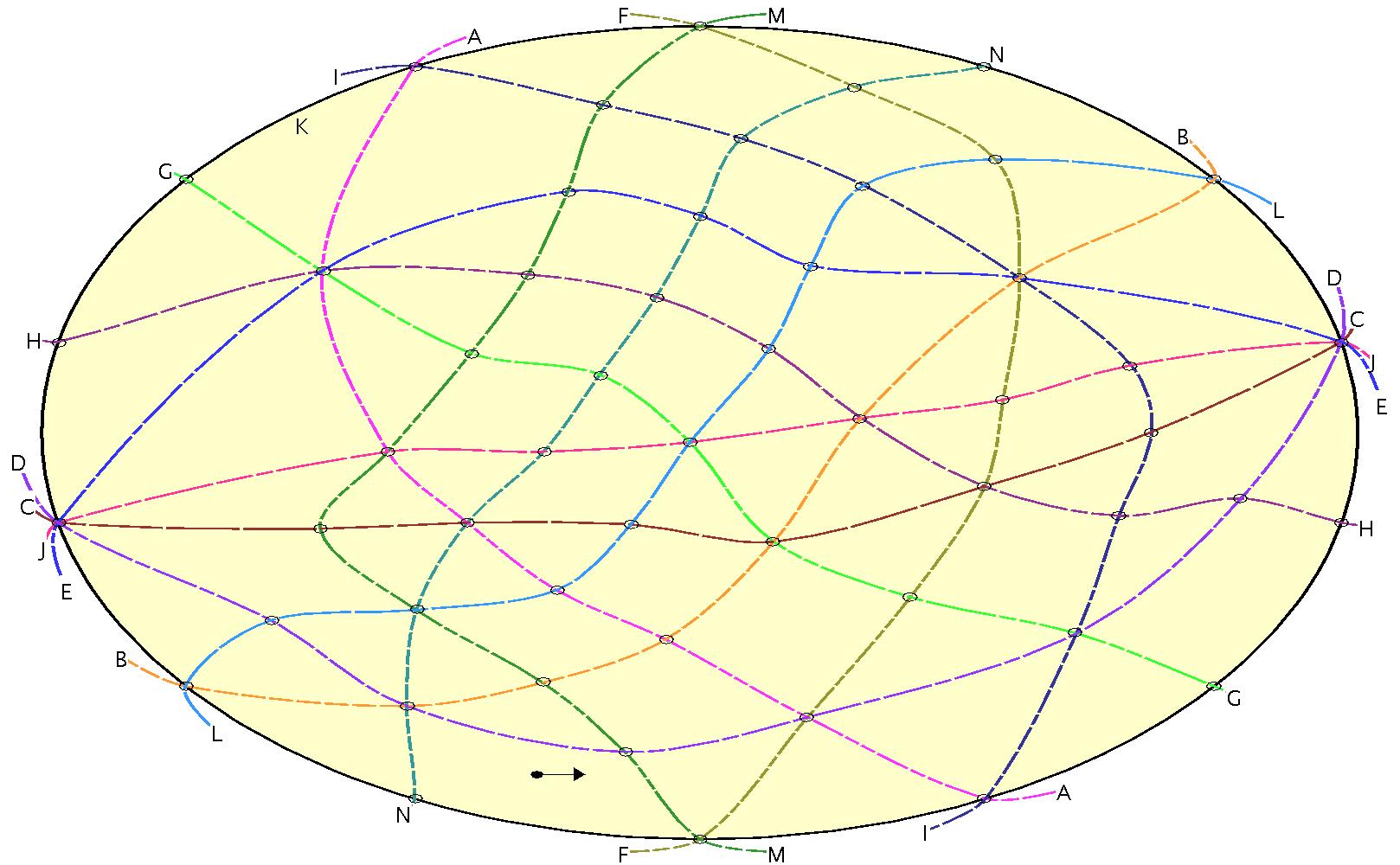
Richter-Gebert, [1996] presents two oriented matroids, both derived from a third. His title, two interesting oriented matroids, suggests he sees the third one as dull — we include it nevertheless. The basic derivation is from this matrix:
| A | B | C | D | E | F | G | H | I | J | K | L | M | N |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 1 | 0 | 1 | 1 | 2 | 3 | 2 | 3 | 1 | 1 | -1 | 0 |
| 0 | 1 | 0 | 1 | 1 | 2 | 1 | 2 | 3 | 1 | 3 | -1 | 1 | 0 |
| 0 | 0 | 1 | 1 | 2 | 2 | 2 | 4 | 4 | 4 | 4 | 4 | 4 | 1 |
This is the boring one of the three, the oriented matroid is taken directly from the matrix. i.e. the vectors of the oriented matroid are the signed dependencies between the columns of the matrix.
B, D, M
F, M< I, A< G< H< E, J, C, D< L, B< N